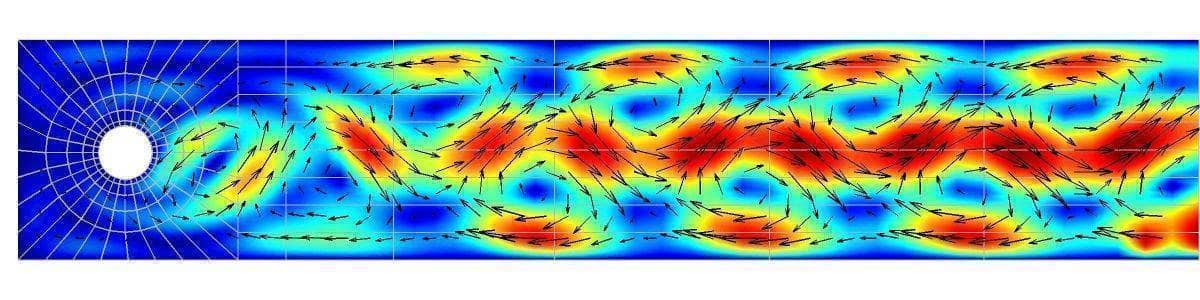
In computational fluid dynamics (CFD) simulations accuracy is often very important, especially for drag and lift values. References [1-2] describe a CFD benchmark problem for time-dependent flow around a cylinder where the Reynolds number varies between 0<=Re<=100. The references contain very accuratly established values for the drag and lift coefficients, and pressure difference between the front and rear of the cylinder.
This benchmark problem has been simulated with FEATool with several grid and time step sizes. The results are shown in the table below.
| L | dt | t(cd_max) | cd_max | t(cl_max) | cl_max | dp(t=8) |
|---|---|---|---|---|---|---|
| 1 | 0.0500 | 3.95000 | 3.04492 | 7.45000 | 0.10927 | -0.10132 |
| 1 | 0.0250 | 3.95000 | 3.04501 | 7.07500 | 0.16774 | -0.10960 |
| 1 | 0.0125 | 3.93750 | 3.04503 | 6.97500 | 0.18064 | -0.10309 |
| 2 | 0.0500 | 4.00000 | 3.07431 | 0.40000 | 1.45990 | -0.10323 |
| 2 | 0.0250 | 4.12500 | 3.07118 | 5.00000 | 0.60703 | -0.10315 |
| 2 | 0.0125 | 3.93750 | 2.95171 | 5.92500 | 0.47294 | -0.10948 |
| 3 | 0.0500 | 3.95000 | 2.94952 | 6.00000 | 0.34591 | -0.09935 |
| 3 | 0.0250 | 3.95000 | 2.94989 | 5.80000 | 0.44944 | -0.09830 |
| 3 | 0.0125 | 3.93750 | 2.95003 | 5.73750 | 0.47833 | -0.10821 |
| 4 | 0.0500 | 3.95000 | 2.95015 | 6.00000 | 0.34475 | -0.10268 |
| 4 | 0.0250 | 3.95000 | 2.95053 | 5.77500 | 0.44271 | -0.10175 |
| 4 | 0.0125 | 3.93750 | 2.95068 | 5.71250 | 0.47006 | -0.11057 |
| Ref. | 3.93625 | 2.95092 | 5.69250 | 0.47835 | -0.11162 | |
As can be seen the computed values on the finest grid and time step are very accurate and close to the reference solution. The time evolution of the lift coefficient is the most difficult quantity to capture correctly, but from the figure below the computed solution is again very close to the reference.
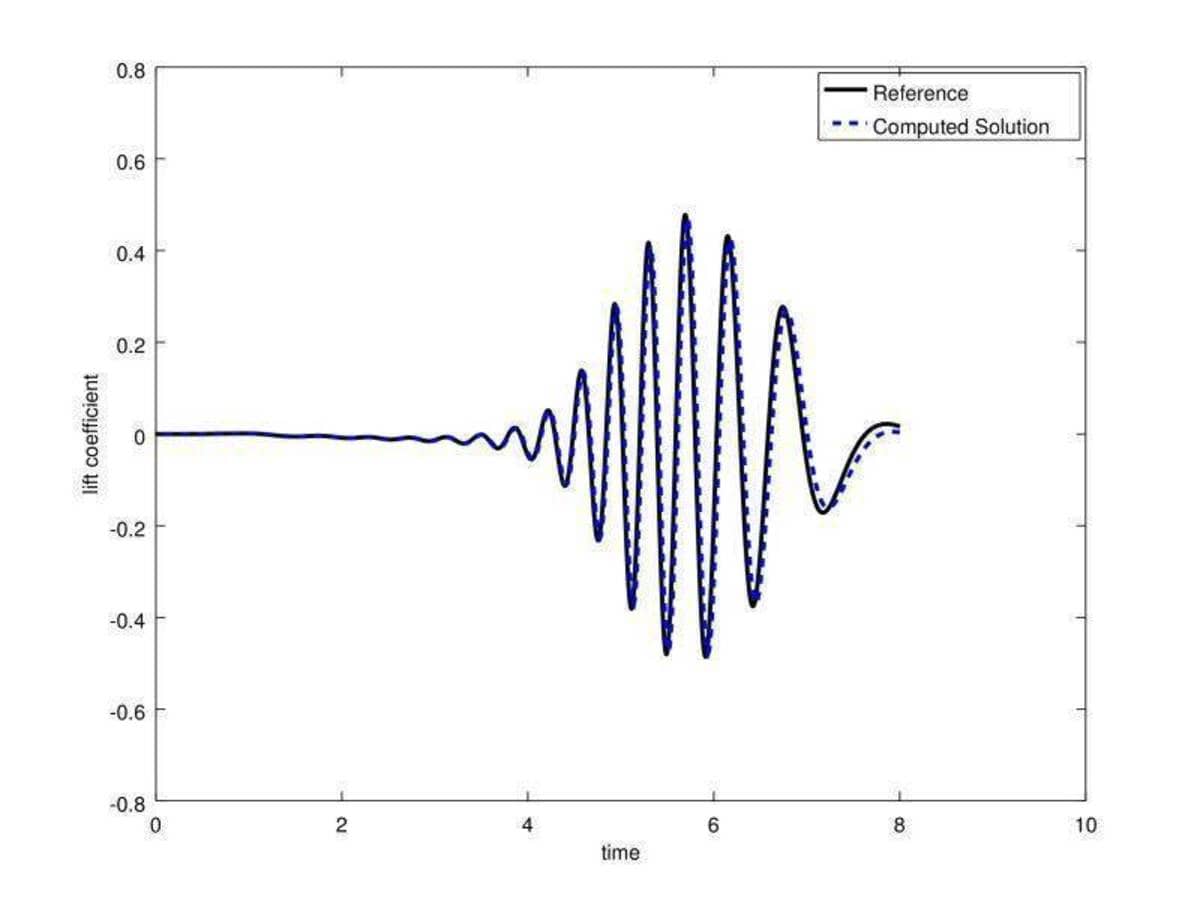
The FEATool model script file to run the time-dependent cylinder benchmark can be found in the FEATool examples directory or from the link below. Note that it might take quite some time for the simulation to finish depending on the choice of grid and time step sizes.
References
[1] John V. Reference values for drag and lift of a two-dimensional time-dependent flow around a cylinder. International Journal for Numerical Methods in Fluids 2004; 44:777-788.
[2] John V, Rang J. Adaptive time step control for the incompressible Navier–Stokes equations. Comput. Methods Appl. Mech. Engrg. 199 (2010) 514–524.